A Newsvendor Formulation of the Army and Army Reserve

1. Problem Statement
   
2. Background and Justification
   
3. Model
   
4. Analysis
   
5. Worth of Project
   

The Army Reserve can be thought of as a strategic reserve for the Army.

• Soldiers voluntarily join the Army Reserve
  
• Work/train 38 days per year
• Receive some benefits
  
• Work a regular job
  
• Could be called into full time service

A relatively inexpensive inventory of soldiers ready for action

Question: How many troops should be in the Army Reserve?

Answer:

Question: How many troops should be in the Army Reserve?

Answer: Develop an inventory model to aide in this decision.

Army Reserve:

• Began in 1908
• 205,000 strong (compare to 541,000 in the Army)
• Can be called to active duty
• Work 38 days a year
  
• $3,216 per year
  

Whats the right size for the US army?

• Current budget proposal calls for reduction
  
• Defense analyst thinks the size should increase
  

• We can use an inventory model to find the economically optimal decision.
  
• This is crucial for fiscal responsibility
  

Newsvendor:

• Products lose value after time
• Only one opportunity to place an order
  
• Distribution of demand
  
• Holding cost
  
• Shortage cost

Newsvendor meets Army Reserve

Products lose value after time:

• Soldiers have a limited shelf life. (Assume 8 years)
• How many old soldiers do you know?
  

Newsvendor meets Army Reserve

Products lose value after time:

• Soldiers have a limited shelf life. (Assume 8 years)

• How many old soldiers do you know?

  

Newsvendor meets Army Reserve

Only one opportunity to place an order:

• Assume they place an order every 8 years
  
• This is a simplifying assumption
  
• How many would sign up during war?

Newsvendor meets Army Reserve

Only one opportunity to place an order:

• Assume they place an order every 8 years
  
• This is a simplifying assumption
  
• How many would sign up during war?
  

Newsvendor meets Army Reserve

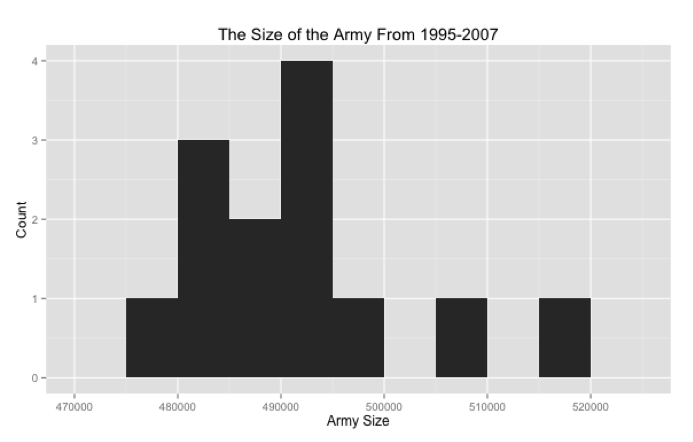
Distribution of demand:

• Historical data
  
  

Newsvendor meets Army Reserve

Holding cost:

• Cost to recruit ($15,000)
  
• Cost to pay ($3,216 a year)

Newsvendor meets Army Reserve

Shortage cost:

• Unknown
  
• Loss of life and loss of freedoms
• Can make a guess through analysis

Calucluate Shortage Cost

• Known demand distribution
  
• Assume current troop level (S) is optimal
  
• \( S^*=F^{-1}\left(\frac p{h+p} \right) \)
  
• \( p=\frac{hF(S)}{1-F(S)} \)

\( \mu=541,291 \)
\( \sigma=17,134 \)
\( S=746,291 \)
\( h=40,728 \ dollars \)

\( p=\frac{hF(S)}{1-F(S)}=Infinity \)

\( Type \ I \ service \ level=100\% \)

\( Type \ II \ service \ level=100\% \)

\( \mu=541,291 \)
\( \sigma=17,134 \)
\( S=746,291 \)
\( h=40,728 \ dollars \)

\( p=\frac{hF(S)}{1-F(S)}=Infinity \)

\( Type \ I \ service \ level=100\% \)

\( Type \ II \ service \ level=100\% \)

Obviously troop levels are not determined with an economic model (or we have the wrong model)

\( \mu=541,291 \)
\( \sigma=17,134 \)
\( S=643,791 \)
\( h=81,456 \ dollars \)

\( p=\frac{hF(S)}{1-F(S)}=7.4 \ trillion \ dollars \)

\( Type \ I \ service \ level \approx 100\% \)

\( Type \ II \ service \ level \approx 100\% \)

\( \mu=541,291 \)
\( \sigma=17,134 \)
\( S=643,791 \)
\( h=81,456 \ dollars \)

\( p=\frac{hF(S)}{1-F(S)}=7.4 \ trillion \ dollars \)

\( Type \ I \ service \ level \approx 100\% \)

\( Type \ II \ service \ level \approx 100\% \)

Refinements must be made to the model

Allow the decision to be made on service level

Type I:
\( \mu=541,291 \)
\( \sigma=17,134 \)
\( \alpha=0.999 \)
\( h=81,456 \ dollars \)

\( S^{\alpha}=F^{-1}\left(\frac {\frac{h\alpha}{(1-\alpha)}}{h+\left(\frac{h\alpha}{(1-\alpha)} \right)} \right) = 594,239 \)

\( Reserve=52,948 \)

Allow the decision to be made on service level

Type I:
\( \mu=541,291 \)
\( \sigma=17,134 \)
\( \alpha=0.999 \)
\( h=81,456 \ dollars \)

\( S^{\alpha}=F^{-1}\left(\frac {\frac{h\alpha}{(1-\alpha)}}{h+\left(\frac{h\alpha}{(1-\alpha)} \right)} \right) = 594,239 \)

\( Reserve=52,948 \)
Demand met for 99.9% of planning periods

Allow the decision to be made on service level

Type II:
\( \mu=541,291 \)
\( \sigma=17,134 \)
\( \beta=0.999 \)
\( h=81,456 \ dollars \)

\( S^{\beta}=\sigma L^{-1}\left(\frac{\mu \left(1-\beta \right)}{\sigma} \right)+\mu=566,820 \)

\( Reserve=25,529 \)

Allow the decision to be made on service level

Type II:
\( \mu=541,291 \)
\( \sigma=17,134 \)
\( \beta=0.999 \)
\( h=81,456 \ dollars \)

\( S^{\beta}=\sigma L^{-1}\left(\frac{\mu \left(1-\beta \right)}{\sigma} \right)+\mu=566,820 \)

\( Reserve=25,529 \)
99.9% of demand met

• Prelim results indicate the Army may be too large
  
• Obviously the inventory model needs to be refined
  
• Similar techniques could be used to aide decision making
  

Walter Bennette
bennette@iastate.edu
bennette.github.io